Literal Functions and Formulas

INTRODUCTION

[3.6] Literal Functions and Formulas

Directions: Find the perimeter of this rectangle.

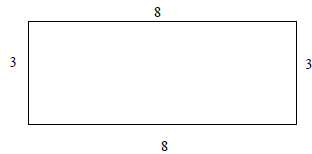
Answer: 8 + 3 + 8 + 3 = 22

“Now let’s represent each side with a variable and make a formula for the perimeter of a
any rectangle. Let’s just replace the numbers with a variable.”

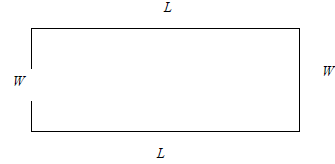
“So L is the length of the rectangle and W is the width of the rectangle. How do we find
the perimeter now? We just add the sides like we did in the previous example.”

P (the perimeter) = L + W + L + W

“Now combine like terms.”

P = 2L + 2W

“This is an example of a literal equation.”

LESSON NOTES

Literal Equation – an equation that uses more than one letter as a variable.

• You can solve for any of the variables in the equation
• Solve these equations the same way you solve equations with one variable
• Want to get one variable by itself

“Suppose you are asked to find the width of the rectangle above. So we need to find W.
How do we do this?”

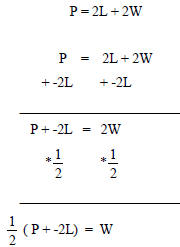	“Add the opposite”
	“Multiply by the reciprocal”

“So you have now solved this equation for W.”

• Process: SADMEP

1. Subtract / Add any numbers or other variables (Do the opposite)
2. Divide / Multiply any numbers or other variables (Reciprocalize)

EXAMPLE PROBLEMS

Example #1

Solve for b.

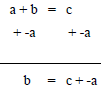“Add the opposite of a”

Example #2

5x + y = 2

Solve for x.

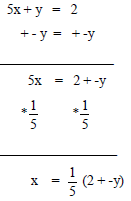	“Add the opposite of y”
	“Multiply by reciprocal”

Solve for y.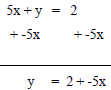

“Add the opposite of 5x”

Example #3 (slope-intercept form)

Solve for m.		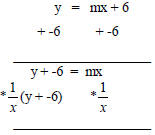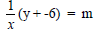“Add the opposite of 6”
		“Multiply by the reciprocal of x”

Example #4

Solve for R. A = R – tR

“We need R, so we need to undistribute.”

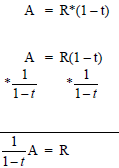	“It may be helpful to think of this as A = 1R – tR”
	“It may be helpful to think of this as A = 1R – tR”

HOMEWORK (write on board)

Homework: Page 157 - # 1 – 12, 19 – 20

DUE Tomorrow, October 31, 2007

[3.6] Literal Functions and Formulas

Tomorrow is Halloween and you want to know how hot or cold it will be. However,
when you look up the weather, you see that is it written in °C.

Wednesday, October 31, 2007 High: 10°C Low: 5°C

Part A: Solve the following literal equation for °F.

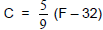
Part B: Using your answer from Part A, find what the high and low temperatures on
Halloween will be in °F.

High:

Low: