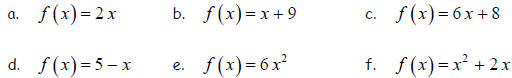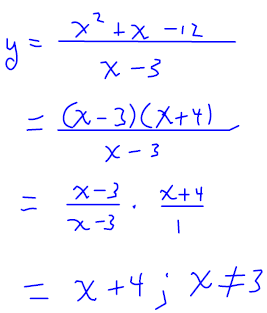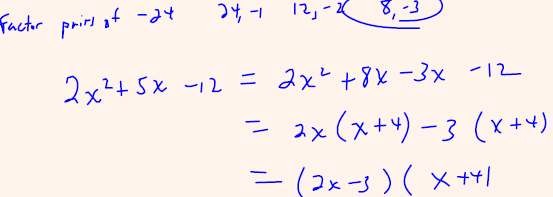Introduction to rational functions
Textbook sections and practice problems
6.1: 1 – 10 all, 13, 15, 25-59 odd, 93, 97, 95 |
Definition
A function f whose formula can be written in the form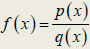
 where p and q are
both polynomial functions is called a rational function. |
Example 1
Graph the function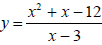 
on your calculator and
carefully copy the graph onto Figure 1.
State the domain of the function. Finally, write the equation for the function
in simplified form.
| Recall
To factor x2 + x − 12 we first
need to find an
integer factor pair of −12 that adds to 1. |
|
 |
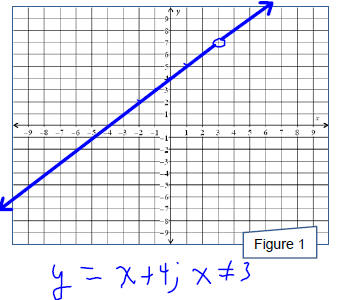Example 2
Graph the function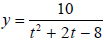
on your calculator and
carefully copy the graph onto Figure 2.
State the domain of the function. Finally, write the equation for the function
in simplified form.
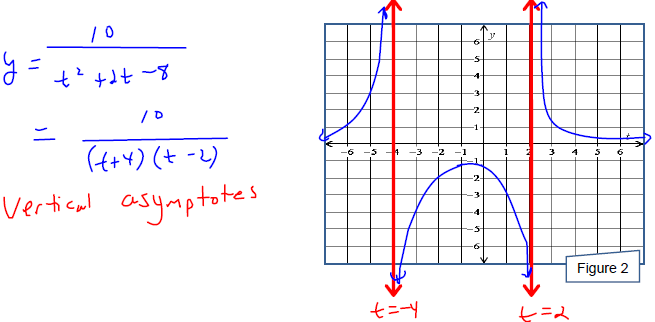
Recall
To factor t2 + 2t − 8 we first need to find an integer factor
pair of −8 that adds to 2 . |
Example 3
Simplify the formula for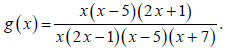 
Make sure that you
state any
necessary domain restrictions. State any other numbers that are not in the
domain of g .
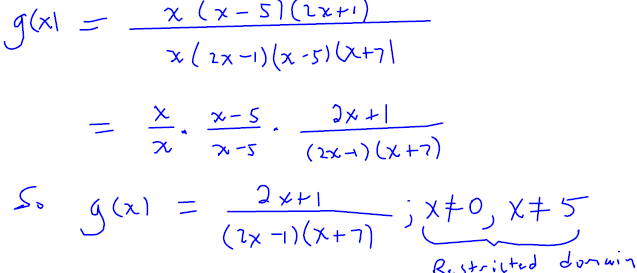
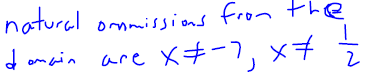
Example 4
Simplify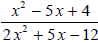 
. Make sure that you state any
necessary domain restrictions.
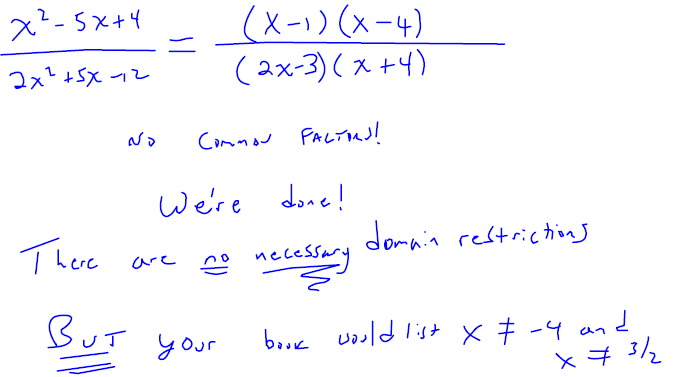
Recall
To factor x2 − 5 x + 4 we first need to find an integer
factor pair of 4 that adds to −5
To factor 2 x2 + 5 x −12 we first need
to find an integer factor pair of (2)(−12) that adds to 5
|
Example 4
Simplify each rational expression; make sure that you state any necessary
restrictions to the
domains.
Simplify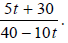 
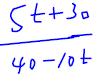
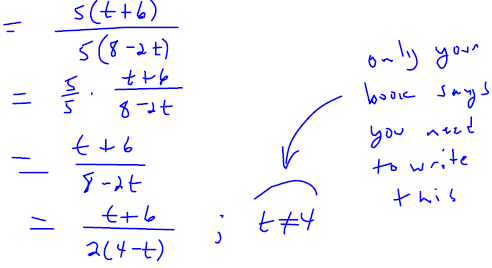
Simplify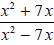 
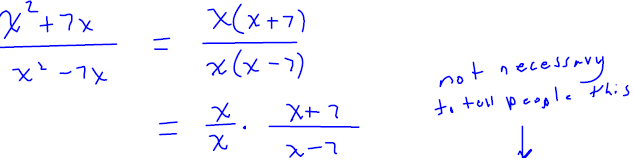
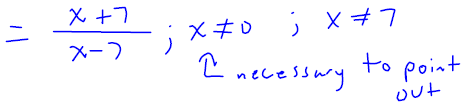
Simplify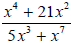 
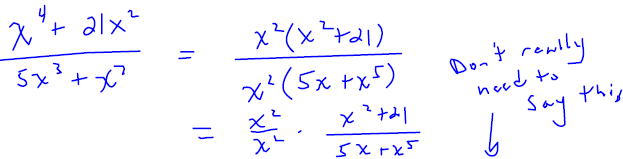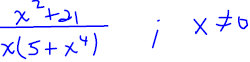Example 5
Simplify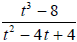 
. State any necessary restrictions
on the domain.
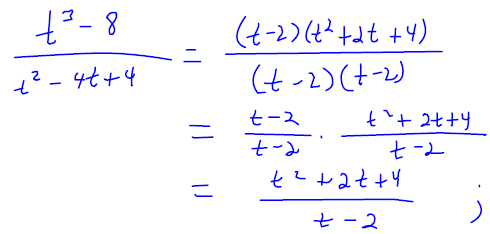
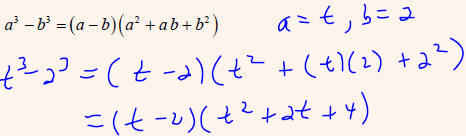Example 6
Simplify the formula for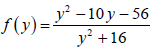
. What is the domain
of f ?
 |
 |
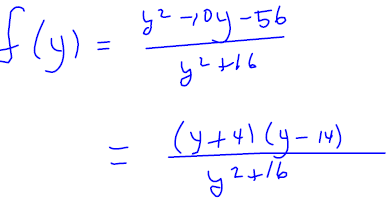Thinking about what y couldn't be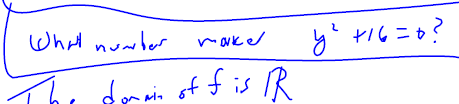
 |
 |
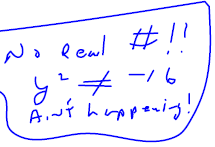a2-b2=(a-b) (a+b)
a2+b2 is psime! |
Example 7
Simplify the formula for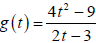
. State any necessary
restrictions on the domain. What
other numbers are not in the domain of g ?
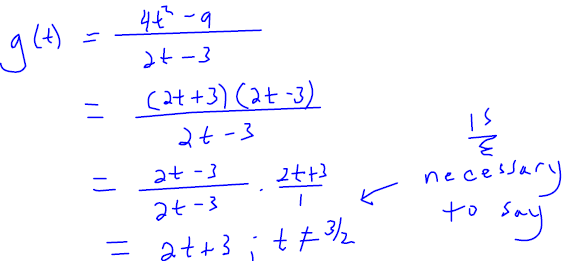
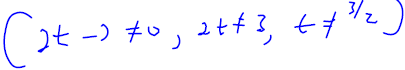
Example 8
Simplify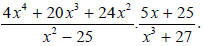
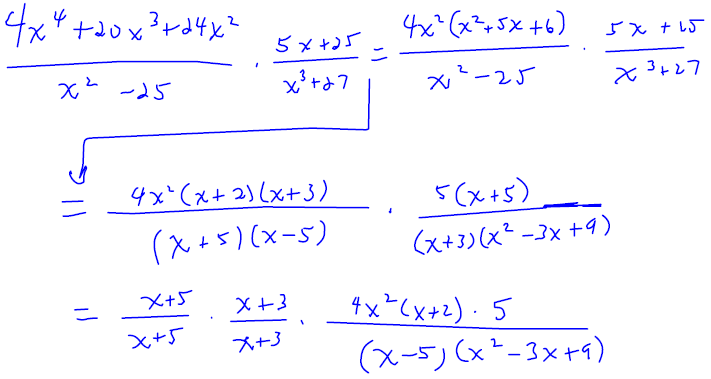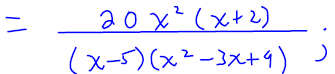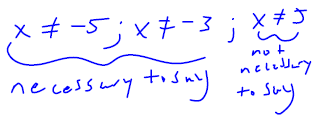Example 9
Suppose that f (x) = 3 x + 2 , g (t ) = 2 − 7t , and m(x) = x2
a. Find and simplify f (x + h) , g (t + h) , and m(x + h) .
b. Find and simplify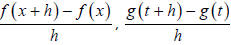 
, and
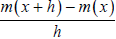
Additional practice problems for you
1. Complete simplify each expression. Make sure that you state all necessary
domain restrictions.
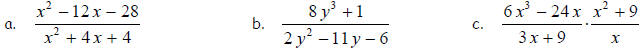
2. Simplify each function formula making sure that you
state any necessary domain restrictions.
Then state the domain of the function using interval notation.
3. Find and completely simplify f (x + h) for each of the
following function.
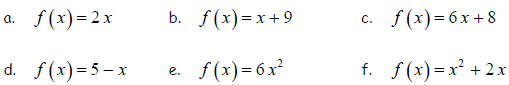
4. Find and completely simplify
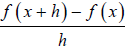for each of the following function.
